Â鶹ÒùÔº

Kirigami is a traditional Japanese art form that entails cutting and folding paper to produce complex three-dimensional (3D) structures or objects. Over the past decades, this creative practice has also been applied in the context of physics, engineering, and materials science research to create new materials, devices and even robotic systems.

Researchers at Sichuan University and McGill University recently devised a new approach for the inverse engineering of kirigami, which does not rely on advanced computational tools and numerical algorithms. This new method, outlined in a paper in Â鶹ÒùÔºical Review Letters, could simplify the design of intricate kirigami for a wide range of real-world applications.

"This work is a natural extension of our previous work on kirigami," Damiano Pasini, senior corresponding author of the paper, told Â鶹ÒùÔº.

"The first author, Chuan Qiao, is a former Ph.D. student of mine. During his Ph.D. under my supervision, Chuan worked on snapping interaction of thin shells and anisotropic morphing of kirigami. The study of the latter led to , where we adopted a unit cell approach to investigate the deformation of a kirigami made of rotating units with arbitrarily shaped triangles."

In their previous studies, the researchers were able to better understand the role that the geometric parameters of slit patterns (e.g., internal angles and lengths) play in the anisotropic deployment of kirigami. To do this, they assessed changes in shape observed in the final deployment of kirigami by examining the length of the sides and internal angles of the triangular units.

"This triggered a set of interrelated questions, such as: how would a kirigami deform if we simply change the entire shape of the rotating unit in its entirety, as opposed to changing its geometric constituents individually, as we did in previous work? Is there a physical relation between the kirigami deformation and the shape of its rotating unit? And can this geometric relation establish an intuitive first-hand route for the inverse design of kirigami?" said Pasini.

"With these questions in mind, our goal then became twofold: to unveil the fundamental relation between the shape of the rotating units and the shape of the deployed kirigami and to leverage such a relation for the design of kirigami that foregoes the use of fairly sophisticated numerical methods currently used in the literature."

In their new paper, Pasini and his colleagues showed that there is a simple relationship between the strain applied to a rotating unit (γ^*_max) and the shear deformation of a deployed triangle kirigami (γ_max). Their proposed method to inversely design kirigami leverages this straightforward relationship.

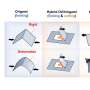
"The key notion is that the shear strain applied to the initial rotating units, which shrink horizontally when we move from the left to the middle sketch, is in the opposite direction of the shear strain of the deployed triangle kirigami, which, in contrast, expands horizontally (once it deploys)," said Pasini.

"This insight can be leveraged for the inverse design of kirigami. By a simple geometric tuning of the shape of the rotating units with shear strains, we can achieve on-target deployment. The only basic operation we need is to use an area-preserving map to apply the shear strain."

The new approach for the engineering of kirigami introduced by this team of researchers requires three key ingredients. These are the contracted shape of the rotating units, the shape of a deployed kirigami, and an area-preserving map outlining the transition from the deployed kirigami to its non-deployed (i.e., contracted) form.

"With the ingredients above, the shear strain of the deployed triangle kirigami can be programmed by applying a shear strain to the shape of the rotating units in the opposite direction," said Pasini. "Our method can now forego any numerical computations and program the shear deformation of a kirigami specimen at will in a swift, versatile manner."

To demonstrate the potential of their inverse design method, Pasini and his colleagues used it to produce three different types of morphing targets, namely the contracted shape, the deployed shape, and the internal trajectories of rotating units in kirigami.

This recent study shows a fundamental relationship between the deformation of kirigami and the shape of their rotating units, which can be utilized to design these structures. The team's geometric design method could soon be used to create a wide range of kirigami designs that could help to tackle complex engineering challenges.

"This work brings ground-rule insights into morphing matter with rotating units and offers an intuitive, firsthand geometric route for the swift design of complex kirigami," added Pasini. "Similar observations can also be drawn by inspecting the morphing of quadrilateral kirigami, hence showing the promise of this work to describe other kirigami patterns."

© 2025 Science X Network