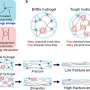
A new law gives the energy needed to fracture stretchable networks

Interconnected materials containing networks are ubiquitous in the world around us—, , human and engineered tissues, woven sheets and chain mail armor. Engineers often want these networks to be as strong as possible and to resist mechanical fracture and failure.
The key property that determines the strength of a network is its intrinsic fracture energy, the lowest energy required to propagate a crack through a unit area of the surface, with the bulk of the network falling apart. As examples, the intrinsic fracture energy of polymer networks is about 10 to 100 joules per square meter, 50–500 J/m2 for elastomers used in car tires, while spider silk has an intrinsic fracture energy of 150–200 J/m2.
Until now, there has been no way to calculate the intrinsic fracture energy (IFE) for a networked material, given the mechanical behavior and connectivity of its constituents.
in the journal Â鶹ÒùÔºical Review X, scientists from the U.S. have developed a scaling law that predicts the IFE of a wide type of stretchable networks. The IFE depends only on the properties of individual strands in the network—how much force is required to break a strand, the length of a strand when it reaches the breaking point—and the network's geometry—how many strands there are per unit area.
The IFE is then proportional to the product of these three quantities. Their result is backed up by experiments and simulations to "a breadth of strand constitutive behaviors, topologies, dimensionalities, and length scales," they write, "including but not limited to polymer-like networks."
The result applies across multiple length scales from a nanometer to a meter and works for a span of two- and three-dimensional network architectures, such as triangular networks, square networks, hexagonal networks, networks that are body-centered cubic, and cubic lattices.
To develop the new law for intrinsic fracture energy, the group from universities in Massachusetts and Georgia in the United States with a co-author from Inkbit Corporation in Massachusetts, directly assembled networks of many materials and carefully tested them.
They began analyzing various network geometries by considering individual strands of an initial length, a final length at the breaking point, and the strand's rupture force. These behaviors could be linear or nonlinear. Networked materials were fabricated by layering the strands; there could be as many as a few thousand layers for each network.
They started with an equation that had been developed in the Â鶹ÒùÔºical Properties of Polymers by Frederick J. Bueche, which was modified in 1996 for a on the elastic properties of DNA. Both were based on a model of a polymer chain called the freely jointed chain model, which is a chain of statistically independent Kuhn segments of equal length whose segment orientations are uncorrelated in the absence of external forces.
Kuhn segments are an idealized segment of a polymer chain whose joints (to its neighboring segments) are free to align independently (again, the absence of an external force such as a magnetic or electric field).
Modifying the model for experimental testing and validation, they used several materials having a range of two-dimensional and three-dimensional networks created with a laser cutter. Tetra-poly(ethylene glycol) hydrogels (Tetra-PEG hydrogels) especially received attention in their paper. This hydrogel possesses relatively homogeneous networks, with a diamond cubic lattice network architecture.
An universal testing machine was used to restrain the polymer network at one end, then pull with a force at the other end, until the network tore.
Besides laboratory experiments, "we developed a coarse-grain-based simulation tool," said Bolei Deng from the Georgia Institute of Technology. The coarse-grained method, which leaves the strands with a rough, coarse texture, reconstructed large networks with drastically fewer degrees of freedom—the number of ways the interconnected polymer chains can move.
In one coarse-grained triangular network created for simulations, the network had 4,000 vertical layers and 8,000 horizontal layers, with a total of 44,847 nodes and 89,694 degrees of freedom. The simulations "allow us to simulate the fracture energy of extremely large networks with thousands of layers with minimal computational cost and visualize the energy flow during the fracture process," Deng said.
Potential applications for architected materials, where structures and networks within the material give it unique properties, include soft robotic actuators, enhancing the toughness and durability of engineered tissues, and the creation of resilient lattices for aerospace technologies.
"This scaling law provides a roadmap for developing tough, stretchable networks from the ground up," said lead author Chase Hartquist, a Ph.D. candidate in mechanical engineering at the Massachusetts Institute of Technology.
"Instead of relying on intuition, scientists and engineers can use these findings to intentionally design and directly construct network materials with targeted performance."
More information: Chase Hartquist et al, Scaling Law for Intrinsic Fracture Energy of Diverse Stretchable Networks, Â鶹ÒùÔºical Review X (2025).
Journal information: Â鶹ÒùÔºical Review X
© 2025 Science X Network